Point (geometry): Difference between revisions
imported>Joe Quick m (sp) |
mNo edit summary |
||
| (24 intermediate revisions by 7 users not shown) | |||
| Line 1: | Line 1: | ||
A '''point''' is a | {{subpages}} | ||
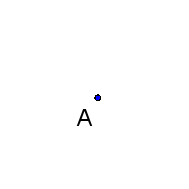
[[Image:Point (geometry).png|frame|Diagram of a point (in blue) called A (with its label in black)]] | |||
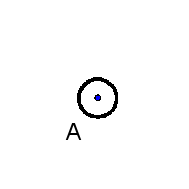
[[Image:Point (geometry) circle notation.png|frame|Diagram of a point using an alternative "circle" notation]] | |||
A '''point''' is a mathematical object in [[geometry]] which has a position but neither [[length]] nor [[breadth]] nor [[depth]]. | |||
A point is made up of no other parts. | A point is made up of no other parts. | ||
The extremities of a [[line (geometry)|line]] are points. | The extremities of a [[line (Euclidean geometry)|line]] segment are points. | ||
A [[line (geometry)|line]] is made up of an infinite number of points. | Geometric objects, such as lines, planes, line segments, triangles, circles, disks, spheres, balls, cubes etc., are often (but not always) treated as [[set (mathematics)|sets]] of points. | ||
Any [[line (geometry)|line]] can be completely defined by two distinct points. | |||
Any [[plane (geometry)|plane]] can be completely defined by three distinct points that are not all part of the same [[line (geometry)|line]]. | In [[Euclidean geometry]]: | ||
Any two [[line (geometry)|lines]] that lay on a single [[plane (geometry)|plane]] and are not [[parallel (geometry)|parallel]] | |||
A [[line (Euclidean geometry)|line]] is made up of an infinite number of points. | |||
[[Category: | Any [[line (Euclidean geometry)|line]] can be completely defined by two distinct points. | ||
Any [[plane (geometry)|plane]] can be completely defined by three distinct points that are not all part of the same [[line (Euclidean geometry)|line]]. | |||
Any two [[line (Euclidean geometry)|lines]] that lay on a single [[plane (geometry)|plane]] and are not [[parallel (geometry)|parallel]] (nor coincide) intersect at a single point. | |||
Three planes typically intersect at a single point (though in special cases their intersection can be [[empty set|empty]], a line, or a plane). | |||
==Beyond mathematics== | |||
{{Image|847908976 22a19746b6.jpg|right|200px|[http://www.flickr.com/photos/77945684@N00/847908976/ Stubby marker], a high-accuracy geodetic control mark.}} | |||
In geodesy, a point on the Earth is marked by a so-called geodetic control mark. The distance between such points can be determined with the accuracy about 10<sup>-7</sup> of the distance (say, 100 km plus/minus 10 mm). | |||
Individual atoms are probably the smallest (about 10<sup>–10</sup> m) marks on a surface that persist during a macroscopic time (hours, days). See [http://picasaweb.google.com/IBMResearchAlmaden/IBMCelebrates20YearsOfMovingAtoms#5385521934130340162 the world’s smallest corporate logo].[[Category:Suggestion Bot Tag]] | |||
Latest revision as of 06:01, 5 October 2024
A point is a mathematical object in geometry which has a position but neither length nor breadth nor depth. A point is made up of no other parts. The extremities of a line segment are points. Geometric objects, such as lines, planes, line segments, triangles, circles, disks, spheres, balls, cubes etc., are often (but not always) treated as sets of points.
A line is made up of an infinite number of points. Any line can be completely defined by two distinct points. Any plane can be completely defined by three distinct points that are not all part of the same line. Any two lines that lay on a single plane and are not parallel (nor coincide) intersect at a single point. Three planes typically intersect at a single point (though in special cases their intersection can be empty, a line, or a plane).
Beyond mathematics
In geodesy, a point on the Earth is marked by a so-called geodetic control mark. The distance between such points can be determined with the accuracy about 10-7 of the distance (say, 100 km plus/minus 10 mm).
Individual atoms are probably the smallest (about 10–10 m) marks on a surface that persist during a macroscopic time (hours, days). See the world’s smallest corporate logo.