Spherical harmonics: Difference between revisions
imported>Paul Wormer m (wikilink) |
mNo edit summary |
||
| (34 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
{{subpages}} | {{subpages}} | ||
{{TOC|right}} | |||
*''See [[Spherical harmonics/Catalogs]] for a table of spherical harmonics through ℓ'' = 4. | |||
'''Spherical harmonics''' are functions arising in [[physics]] and [[mathematics]] when [[spherical polar coordinates]] (coordinates ''r'', θ and φ that locate a point in space) are used in investigating physical problems in three dimensions. The functions appear in physical problems with (near-) spherical symmetry, indeed, in the same kind of physical problems where spherical polar coordinates are preferred over other coordinate systems such as [[Cartesian coordinates|Cartesian]] or [[cylinder coordinates|cylinder]] coordinates. | |||
The name "spherical harmonics" was first used by [[William Thomson]] (Lord Kelvin) and [[Peter Guthrie Tait]] in their 1867 ''Treatise on Natural Philosophy''.<ref>See N. M. Ferrers, ''An Elementary Treatise on Spherical Harmonics'', MacMillan, 1877 (London) p. 3 [http://www.archive.org/stream/elementarytreati00ferriala#page/2/mode/2up Online].</ref> The term ''harmonic function'' was coined earlier by William Thomson for solutions of the [[Laplace equation]], ∇²''V'' = 0, and as the spherical harmonic functions appear as the solution of the Laplace equation in spherical polar coordinates, their name followed immediately. In German the functions are called "Kugelfunktionen" (literally sphere functions), and in French they are known as "fonctions harmoniques sphériques", which is equivalent to their English name. | |||
In [[quantum mechanics]] spherical harmonics appear as eigenfunctions of (squared) [[Angular momentum (quantum)#Orbital angular momentum|orbital angular momentum]]. Spherical harmonics are ubiquitous in atomic and molecular physics. Further, they are important in the representation of the gravitational and magnetic fields of planetary bodies, the characterization of the cosmic microwave background radiation, the rotation-invariant description of 3D shapes in computer graphics, the description of electrical potentials due to charge distributions, and in certain types of fluid motion. | |||
It can be shown that the spherical harmonics, almost always written as <math>Y^m_\ell(\theta,\phi)</math>, form an orthogonal and complete set (a basis of a [[Hilbert space]]) of functions of the spherical polar angles, θ and φ, with ''ℓ'' and ''m'' indicating degree and order of the function. This implies that the harmonics can be used to describe a function of θ and φ in the form of a linear expansion; the expansion coefficients may be used as [[linear regression]] parameters, which means that they may be chosen such that the original and expanded function "resemble" each other as closely as possible. The more spherical symmetry the original function possesses, the shorter the expansion and the fewer fit (regression) parameters have to be determined. | |||
==Some illustrative images of real spherical harmonics== | ==Some illustrative images of real spherical harmonics== | ||
Polar plots are shown of a few low-order real spherical harmonics (functions of θ and φ) to be defined in this article. The plots show clearly the nodal planes of the functions. The absolute values are meaningless because the functions are not normalized and accordingly the normalization factors are omitted from their definitions. | Polar plots are shown of a few low-order real spherical harmonics (functions of θ and φ) to be defined in this article. The plots show clearly the nodal planes of the functions. The absolute values are meaningless because the functions are not normalized and accordingly the normalization factors are omitted from their definitions. | ||
{{Image|Spherical_harmonics.png|center|700px| }} | |||
==Definition of complex spherical harmonics== | ==Definition of complex spherical harmonics== | ||
The notation <math>Y^m_\ell</math> will be reserved for the complex-valued functions normalized to unity. It is convenient to introduce first non-normalized functions that are proportional to the <math>Y^m_\ell</math>. Several definitions are possible, we start with one that is common in quantum mechanically oriented texts. The [[spherical polar angles]] are the colatitude angle θ and the longitudinal (azimuthal) angle φ. The numbers '' | The notation <math>Y^m_\ell</math> will be reserved for the complex-valued functions normalized to unity. It is convenient to introduce first non-normalized functions that are proportional to the <math>Y^m_\ell</math>. Several definitions are possible, we start with one that is common in quantum mechanically oriented texts. The [[spherical polar coordinates|spherical polar angles]] are the colatitude angle θ and the longitudinal (azimuthal) angle φ. The numbers ''ℓ'' and ''m'' are integral numbers and ''ℓ'' is positive or zero. | ||
:<math> | :<math> | ||
C_\ell^m(\theta,\varphi) \equiv i^{m+|m|}\; \left[\frac{(\ell-|m|)!}{(\ell+|m|)!}\right]^{1/2} P^{(|m|)}_\ell(\cos\theta) e^{im\varphi}, \qquad -\ell \le m \le \ell, | C_\ell^m(\theta,\varphi) \equiv i^{m+|m|}\; \left[\frac{(\ell-|m|)!}{(\ell+|m|)!}\right]^{1/2} P^{(|m|)}_\ell(\cos\theta) e^{im\varphi}, \qquad -\ell \le m \le \ell, | ||
| Line 26: | Line 37: | ||
\tilde{C}_\ell^m(\theta,\varphi) \equiv (-1)^m \left[\frac{(\ell-m)!}{(\ell+m)!}\right]^{1/2} P^{(m)}_\ell(\cos\theta) e^{im\varphi}, \qquad -\ell \le m \le \ell, | \tilde{C}_\ell^m(\theta,\varphi) \equiv (-1)^m \left[\frac{(\ell-m)!}{(\ell+m)!}\right]^{1/2} P^{(m)}_\ell(\cos\theta) e^{im\varphi}, \qquad -\ell \le m \le \ell, | ||
</math> | </math> | ||
The two definitions obviously agree for positive and zero ''m'', but for negative ''m'' this is less apparent. It is also not immediately clear that the choices of phases yield the same function. However, below we will see that the definitions agree for negative ''m'' as well. Hence, for all '' | The two definitions obviously agree for positive and zero ''m'', but for negative ''m'' this is less apparent. It is also not immediately clear that the choices of phases yield the same function. However, below we will see that the definitions agree for negative ''m'' as well. Hence, for all ''ℓ'' ≥ 0, | ||
:<math> | :<math> | ||
\tilde{C}_\ell^m(\theta,\varphi) \equiv C_\ell^m(\theta,\varphi), \quad\hbox{for}\quad m=-\ell,\ldots,\ell. | \tilde{C}_\ell^m(\theta,\varphi) \equiv C_\ell^m(\theta,\varphi), \quad\hbox{for}\quad m=-\ell,\ldots,\ell. | ||
| Line 72: | Line 83: | ||
\int_{0}^{\pi} \int_{0}^{2\pi} C_\ell^m(\theta, \varphi)^* C_{\ell'}^{m'}(\theta, \varphi) \;\sin\theta\, d\theta \, d\varphi = \delta_{\ell\ell'}\delta_{mm'} \frac{4\pi}{2\ell+1}. | \int_{0}^{\pi} \int_{0}^{2\pi} C_\ell^m(\theta, \varphi)^* C_{\ell'}^{m'}(\theta, \varphi) \;\sin\theta\, d\theta \, d\varphi = \delta_{\ell\ell'}\delta_{mm'} \frac{4\pi}{2\ell+1}. | ||
</math> | </math> | ||
The integral over φ gives 2π and a Kronecker delta on | The integral over φ gives 2π and a Kronecker delta on ''m'' and ''m''′. Thus, for the integral over θ it suffices to consider the case ''m'' = ''m'''. The necessary integral is given [[Associated legendre function#Orthogonality relations|here]]. The (non-unit) normalization of <math>\,C^m_\ell</math> is known as [[Racah]]'s normalization or [[Schmidt]]'s semi-normalization. It is often more convenient than unit normalization. Unit normalized functions are defined as follows | ||
:<math> | :<math> | ||
Y_\ell^{m}(\theta,\varphi) \equiv \sqrt{\frac{2\ell+1}{4\pi}} C_\ell^{m}(\theta,\varphi). | Y_\ell^{m}(\theta,\varphi) \equiv \sqrt{\frac{2\ell+1}{4\pi}} C_\ell^{m}(\theta,\varphi). | ||
| Line 78: | Line 89: | ||
==Condon-Shortley phase== | ==Condon-Shortley phase== | ||
One source of confusion with the definition of the spherical harmonic functions concerns the phase factor. In quantum mechanics the phase, introduced above, is commonly used. It was introduced by Condon and Shortley.<ref>E. U. Condon and G. H. Shortley,''The Theory of Atomic Spectra'', Cambridge University Press, Cambridge UK (1935).</ref> In the quantum mechanics community, it is common practice to either include this [[phase factor]] in the definition of the [[associated Legendre function]]s, or to prefix it to the definition of the spherical harmonic functions, as done above. There is no requirement to use the Condon-Shortley phase in the definition of the spherical harmonic functions, but including it can simplify some quantum mechanical operations, especially the application of [[ | One source of confusion with the definition of the spherical harmonic functions concerns the phase factor. In quantum mechanics the phase, introduced above, is commonly used. It was introduced by Condon and Shortley.<ref>E. U. Condon and G. H. Shortley,''The Theory of Atomic Spectra'', Cambridge University Press, Cambridge UK (1935).</ref> In the quantum mechanics community, it is common practice to either include this [[phase factor]] in the definition of the [[associated Legendre function]]s, or to prefix it to the definition of the spherical harmonic functions, as done above. There is no requirement to use the Condon-Shortley phase in the definition of the spherical harmonic functions, but including it can simplify some quantum mechanical operations, especially the application of [[Angular momentum (quantum)#Abstract angular momentum operators|raising and lowering operators]]. The geodesy and magnetics communities never include the Condon-Shortley phase factor in their definitions of the spherical harmonic functions. | ||
==Properties== | ==Properties== | ||
* For ''m'' ≠ 0 the associated Legendre function contains the factor (1−x<sup>2</sup>) and the ordinary Legendre polynomial ''P''<sub>n</sub>(1) = 1. So, | |||
:<math> | ::<math> | ||
C_\ell^m(0,0) = \delta_{m,0} \Longrightarrow Y_\ell^m(0,0) = \delta_{m,0}\,\sqrt{\frac{2\ell+1}{4\pi}}. | C_\ell^m(0,0) = \delta_{m,0} \Longrightarrow Y_\ell^m(0,0) = \delta_{m,0}\,\sqrt{\frac{2\ell+1}{4\pi}}. | ||
</math> | </math> | ||
The regular [[solid harmonics]] ''r''<sup>'' | * The regular [[solid harmonics]] ''r''<sup>''ℓ''</sup>''Y''<sub>''ℓ''</sub><sup>''m''</sup> are homogeneous of degree ''ℓ'' in the components ''x'', ''y'', and ''z'' of '''r''', so that inversion '''r''' → −'''r''' gives the factor (−1)<sup>''ℓ''</sup> for the regular solid harmonics. Inversion of spherical polar coordinates: ''r'' → ''r'', θ → π−θ, and φ → π+φ. So, | ||
θ → π | |||
:<math> | ::<math> | ||
Y_\ell^m(\pi-\theta, \pi+\varphi) = (-1)^\ell Y_\ell^m(\theta, \varphi). | Y_\ell^m(\pi-\theta, \pi+\varphi) = (-1)^\ell Y_\ell^m(\theta, \varphi). | ||
</math> | </math> | ||
* Reflection in the ''x-y'' plane: | |||
:<math> | :<math> | ||
Y_\ell^m(\pi-\theta, \varphi) = (-1)^{\ell-m} Y_\ell^m(\theta, \varphi). | Y_\ell^m(\pi-\theta, \varphi) = (-1)^{\ell-m} Y_\ell^m(\theta, \varphi). | ||
| Line 100: | Line 113: | ||
\mathbf{L} = -i \hbar \mathbf{r} \times \mathbf{\nabla}, | \mathbf{L} = -i \hbar \mathbf{r} \times \mathbf{\nabla}, | ||
</math> | </math> | ||
where the cross stands for the [[cross product]] of the position vector '''r''' and the [[gradient]] ∇; | where the cross stands for the [[cross product]] of the position vector '''r''' and the [[gradient]] ∇; ℏ is [[Planck's constant]] divided by 2π. | ||
The components of '''L''' satisfy the angular momentum [[commutation relations]] | The components of '''L''' satisfy the angular momentum [[commutation relations]]: | ||
:<math> | :<math> | ||
[L_i, L_j] = i\hbar\sum_{j=1}^3 \epsilon_{ijk} L_k,\qquad i,j,k = x,y,z, | [L_i, L_j] = i\hbar\sum_{j=1}^3 \epsilon_{ijk} L_k,\qquad i,j,k = x,y,z, | ||
</math> | </math> | ||
where ε<sub>ijk</sub> is the [[Levi-Civita symbol]]. In [[angular momentum (quantum)|angular momentum theory]] it is shown that these commutation relations are sufficient to prove that ''L''² has eigenvalues '' | where ε<sub>ijk</sub> is the [[Levi-Civita symbol]]. These relations define a [[#Lie algebra|Lie algebra]], as discussed later in this article. In [[angular momentum (quantum)|angular momentum theory]] it is shown that these commutation relations are sufficient to prove that ''L''² has eigenvalues ''ℓ''(''ℓ''+1), | ||
:<math> | :<math> | ||
(L_x^2+L_y^2+L_z^2) \Psi \equiv L^2 \Psi = \hbar^2 \ell(\ell+1) \Psi, | (L_x^2+L_y^2+L_z^2) \Psi \equiv L^2 \Psi = \hbar^2 \ell(\ell+1) \Psi, | ||
</math> | </math> | ||
where | where ''ℓ'' is a [[natural number]]. | ||
From here on we take | From here on we take ℏ equal to unity (this is part of the system of [[atomic units]]). | ||
The operator ''L''² expressed in spherical polar coordinates is, | The operator ''L''² expressed in spherical polar coordinates is, | ||
:<math> | :<math> | ||
| Line 138: | Line 151: | ||
- \frac{m^2}{\sin^2\theta} \right]\Theta(\theta) = 0 . | - \frac{m^2}{\sin^2\theta} \right]\Theta(\theta) = 0 . | ||
</math> | </math> | ||
Upon writing ''x'' = cos θ the equation becomes the [[ | Upon writing ''x'' = cos θ the equation becomes the [[Associated legendre function#Differential equation|associated Legendre equation]] | ||
:<math> | :<math> | ||
(1-x^2) \frac{d^2 \Theta }{dx^2} -2x\frac{d \Theta}{dx} + | (1-x^2) \frac{d^2 \Theta }{dx^2} -2x\frac{d \Theta}{dx} + | ||
| Line 181: | Line 194: | ||
- \frac{L^2}{r^2} \Psi = 0. | - \frac{L^2}{r^2} \Psi = 0. | ||
</math> | </math> | ||
Making the Ansatz Ψ = ''R''(''r'') Y<sup>m</sup><sub> | Making the Ansatz Ψ = ''R''(''r'') Y<sup>m</sup><sub>''ℓ''</sub> the equation becomes | ||
:<math> | |||
\frac{\partial}{\partial r} r^2 \frac{\partial R}{\partial R} = \ell(\ell+1) R, | |||
</math> | |||
where we divided out <math>Y^m_\ell/r^2</math>. Inserting the following functions | |||
:<math> | |||
R_1(r) = r^\ell \quad \hbox{and}\quad R_2(r) = \frac{1}{r^{\ell+1}}. | |||
</math> | |||
shows that these functions are solutions. They give rise to functions known as regular and irregular solid harmonics. See [[solid harmonics]] for more details. | |||
Finally, it is evident that | |||
:<math> | |||
\nabla^2 Y^m_{\ell}(\theta,\phi) = -\frac{\ell(\ell+1)}{r^2} Y^m_\ell(\theta,\phi), | |||
</math> | |||
because <math>\partial Y^m_\ell /\partial r = 0 </math>. | |||
==Lie algebra== | |||
Consider the ''infinitesimal rotation'' from which finite rotations can be generated.<ref name=introduction> | |||
This development is close to that found in {{cite book |title=Quantum field theory demystified |author=David McMahon |url=http://books.google.com/books?id=SoAZVbdyY8AC&pg=PA58 |pages=pp. 58 ''ff'' |chapter=The special orthogonal group SO(N)|publisher=McGraw-Hill Professional |isbn=0071543821 |year=2008}} | |||
</ref> For example, a rotation by angle α about the ''z''-axis is described by the matrix: | |||
:<math>\begin{pmatrix} | |||
\cos \alpha & \sin \alpha & 0\\ | |||
-\sin \alpha & \cos \alpha & 0\\ | |||
0 &0&1 | |||
\end{pmatrix}\ \underset{ \overset{\alpha \rightarrow 0}{}}{\to}\ \begin{pmatrix} | |||
1 & 0 & 0\\ | |||
0 & 1 & 0\\ | |||
0 &0&1 | |||
\end{pmatrix} + i\alpha \begin{pmatrix} | |||
0& -i & 0\\ | |||
i &0 & 0\\ | |||
0 &0&0 | |||
\end{pmatrix} = \begin{pmatrix} | |||
1 & 0 & 0\\ | |||
0 & 1 & 0\\ | |||
0 &0&1 | |||
\end{pmatrix} +i\alpha R_z \ ,</math> | |||
where the form following the arrow applies for very small angles α. The matrix ''R<sub>z</sub>'' is called the ''generator'' of the ''z''-rotation. The factor ''i'' is introduced so the finite rotation can be expressed in terms of this generator as a simple exponential: | |||
:<math>\begin{pmatrix} | |||
\cos \alpha & \sin \alpha & 0\\ | |||
-\sin \alpha & \cos \alpha & 0\\ | |||
0 &0&1 | |||
\end{pmatrix} = e^{i \alpha R_z} \ , </math> | |||
as can be verified using the [[Taylor series]]: | |||
:<math>e^{i \alpha R_z} = 1 + i \alpha R_z + \frac{1}{2} \left( i \alpha R_z\right) ^2 + ... \ . </math> | |||
If the three coordinate axes are labeled {''i, j, k ''} and the infinitesimal rotations about each of these axes are labeled {''R<sub>i</sub>, R<sub>j</sub>, R<sub>k</sub>''}, then these generators of infinitesimal rotations obey the ''commutation relations'':<ref name=Gottfried> | |||
{{cite book |title=Quantum mechanics: fundamentals |author=Kurt Gottfried, Tung-mow Yan |url=http://books.google.com/books?id=8gFX-9YcvIYC&pg=PA77 |pages=p. 77 |isbn=0387955763 |publisher=Springer |edition=2nd ed |year=2003}} | |||
</ref> | |||
:<math> R_i R_j - R_jR_i = i \varepsilon_{ijk} R_k \ , </math> | |||
for any choices of subscripts. Here ε<sub>ijk</sub> is the Levi-Civita symbol mentioned above. The commutation relations express the fact that the order (sequence) of rotations matters. | |||
Suppose these commutation relations now are viewed as applying in general, and while still considered as connected to rotations in three dimensional space, the question is opened as to what general mathematical objects might satisfy these rules. A set of symbols with a defined sum and a product taken as a commutator of the symbols is called a [[Lie algebra]]. <ref name=LieAlgebra>For a mathematical discussion see {{cite book |title=Group Theory: An Intuitive Approach |author=R. Mirman |chapter=§X.7 Angular momentum operators and their algebra |isbn=9810233655 |year=1997 |pages =pp. 292 ''ff'' |url=http://books.google.com/books?id=LFakv848R2oC&pg=PA292 |publisher=World Scientific Publishing Company}} Matrices satisfying the commutation rules are called a ''matrix representation'' of the Lie algebra. See {{cite book |title=Dynamical groups and spectrum generating algebras, vol. 1 |author=BG Adams, J Cizek, J Paldus |editor=Arno Böhm ''et al.'' |edition=Reprint of [http://books.google.com/books?hl=en&lr=&id=sGsR18jJujYC&oi=fnd&pg=PA1 article] in ''Advances in Quantum Chemistry'', vol. 19, Academic Press, 1987 |url=http://books.google.com/books?id=KANTJADFKG4C&pg=PA114 |pages=pp. 114 ''ff'' |chapter= §2.2 Matrix representation of a Lie algebra |isbn=9971501473 |year=1987 |publisher=World Scientific}} | |||
</ref> In particular, one can construct sets of square matrices of various dimensions that satisfy these commutation rules; each set is a so-called ''representation'' of the rules. One finds that there are many such sets, but they can be sorted into two kinds: irreducible and reducible. The reducible sets of matrices can be shown to be equivalent to matrices with smaller irreducible matrices down the diagonal, so that the rules are satisfied within these smaller constituent matrices, and the entire matrix is not essential. The irreducible sets cannot be arranged this way.<ref name=representations> | |||
For a discussion see {{cite book |title=The theory of groups and quantum mechanics |author=Hermann Weyl |isbn=0486602699 |year=1950 |publisher=Courier Dover Publications |edition=Reprint of 1932 ed |url=http://books.google.com/books?id=jQbEcDDqGb8C&pg=PA185 |pages=pp. 185 ''ff'' |chapter=Chapter IV A §1 ''The representation induced in system space by the rotation group''}}, or {{cite book |title =Rotational spectroscopy of diatomic molecules |author=John M. Brown, Alan Carrington |url=http://books.google.com/books?id=TU4eA7MoDrQC&pg=PA143 |chapter=§5.2.4 Representations of the rotation group |pages=pp. 143 ''ff'' |isbn=0521530784 |publisher=Cambridge University Press |year=2003}} | |||
</ref> | |||
The matrices can be viewed as acting upon vectors in an abstract space. For example, a space with an odd number of dimensions (2ℓ+1) can be constructed from the spherical harmonics ''Y''<sub>ℓ</sub><sup>m</sup>, and their transformations under infinitesimal rotations. The ''Y''<sub>ℓ</sub><sup>m</sup> depend upon the angles θ,φ describing orientation in ordinary three-dimensional space, but infinitesimal rotations of these arguments mix up the ''Y''<sub>ℓ</sub><sup>m</sup> in a fashion described by irreducible matrices of dimension (2ℓ+1) that satisfy the commutation relations.<ref name=Hladik> | |||
{{cite book |title=Spinors in physics |author=Jean Hladik |url=http://books.google.com/books?id=25eTtXqLW8UC&pg=PA83 |pages=pp. 83''ff'' |chapter=§3.3.2 Spherical harmonics |isbn=0387986472 |year=1999 |publisher=Springer}} | |||
</ref> | |||
The construction of irreducible matrices of any dimension at all is done as follows. If the generator of an infinitesimal rotation is labeled '''J''', then the basis vectors in this space can be labeled by the integers ''j'' and ''m'' where ''m'' is restricted to the values { −''j'', −''j''+1, ... , ''j''−1, ''j'' }. Denoting a basis vector by |''j, m''⟩, one finds: | |||
:<math>J^2 |j, \ m\rangle = j(j+1) |j, \ m\rangle \ , </math> | |||
:<math>J_z|j, \ m \rangle = m |j, \ m \rangle \ . </math> | |||
Here ''J<sub>z</sub>'' generates an infinitesimal rotation about a direction chosen as the ''z''-axis, and ''J''<sup>2</sup> = ''J<sub>x</sub>''<sup>2</sup> + ''J<sub>y</sub>''<sup>2</sup> + ''J<sub>z</sub>''<sup>2</sup> is the so-called [[Casimir operator]]<ref name=casimir> | |||
{{cite book |title=Groups and Symmetries: From Finite Groups to Lie Groups |author=Yvette Kosmann-Schwarzbach |chapter=§3.2: The Casimir operator |url=http://books.google.com/books?id=8Aa4uS6ZcJEC&pg=PA99 |pages=pp. 99 ''ff'' |isbn=0387788654 |year=2009 |publisher=Springer|edition=Stephanie Frank Singer translation}} | |||
</ref>. In particular, these equations recover the [[Pauli spin matrices]] in two dimensions and the infinitesimal transformations of the ''Y''<sub>ℓ</sub><sup>m</sup> in (2ℓ+1) dimensions.<ref name=Blatt> | |||
For example, see {{cite book |title=Theoretical nuclear physics |author=John M. Blatt, Victor F. Weisskopf |url=http://books.google.com/books?id=R3BzWYQqNGsC&pg=PA782 |pages=p. 782 |isbn=0486668274 |year=1991 |publisher=Courier Dover Publications |edition=Reprint of 1979 Springer-Verlag ed}} | |||
</ref> See the development in [[#Eigenfunctions of orbital angular momentum|Eigenfunctions of orbital angular momentum]] earlier in this article. | |||
==Connection with 3D full rotation group== | ==Connection with 3D full rotation group== | ||
The [[group]] of proper (no reflections) [[rotation]]s in three dimensions is SO(3). It consists of all 3 x 3 [[orthogonal matrix|orthogonal matrices]] with unit [[determinant]]. | The [[group]] of proper (no reflections) [[rotation]]s in three dimensions is SO(3). It consists of all 3 x 3 [[orthogonal matrix|orthogonal matrices]] with unit [[determinant]]. | ||
| Line 192: | Line 294: | ||
\mathcal{R} Y^m_\ell(\hat{\mathbf{r}}) \equiv Y^m_\ell(\mathbf{R}^{-1} \hat{\mathbf{r}}). | \mathcal{R} Y^m_\ell(\hat{\mathbf{r}}) \equiv Y^m_\ell(\mathbf{R}^{-1} \hat{\mathbf{r}}). | ||
</math> | </math> | ||
The inverse matrix appears here (acting on a column vector) in order to assure | The [[inverse matrix]] appears here (acting on a column vector) in order to assure that this map of rotation matrices to rotation operators is a [[group homomorphism]]. Since this point was discussed at some length in [[Wigner]]'s famous book on group theory,<ref>E. P. Wigner, ''Gruppentheorie und ihre Anwendungen auf die Quantenmechanik der Atomspektren'', Vieweg Verlag, Braunschweig (1931). Translated into English: J. J. Griffin, ''Group Theory and its Application to the Quantum Mechanics of Atomic Spectra'', Academic Press, New York (1959).</ref> it is known as ''Wigner's convention''. Some authors omit the inverse on the rotation and find accordingly that the map from matrices to operators is antihomomorphic (i.e., multiplication of operators and matrices is in mutually reversed order). | ||
It can be shown that the rotation operator is an exponential operator in the components of the orbital angular momentum operator '''L'''. It can also be shown that the action of these operators on the spherical harmonics do no change '' | It can be shown that the rotation operator is an exponential operator in the components of the orbital angular momentum operator '''L'''. It can also be shown that the action of these operators on the spherical harmonics do no change ''ℓ''. That is, the linear space spanned by 2''ℓ''+1 spherical harmonics of same ''ℓ'' and different ''m'' is invariant under '''L''', and therefore also under rotations, | ||
:<math> | :<math> | ||
\mathcal{R} Y^m_\ell(\hat{\mathbf{r}}) = \sum_{m'=-\ell}^{\ell} Y^{m'}_\ell(\hat{\mathbf{r}}) | \mathcal{R} Y^m_\ell(\hat{\mathbf{r}}) = \sum_{m'=-\ell}^{\ell} Y^{m'}_\ell(\hat{\mathbf{r}}) | ||
D^{(\ell)}(\mathbf{R})_{m'm}. | D^{(\ell)}(\mathbf{R})_{m'm}. | ||
</math> | </math> | ||
The square | The square 2''ℓ''+1 dimensional matrix that appears here is known as [[Wigner's D-matrix]]. Obviously, the set of matrices of fixed ''ℓ'' form a [[representation]] of the group SO(3). It can be shown that they form an [[irreducible representation]] of this group. The rotation operator is [[unitary]] and the spherical harmonics are [[orthonormal]], hence the Wigner rotation matrix is a unitary matrix: | ||
:<math> | :<math> | ||
\left(\mathbf{D}^{(\ell)}\right)^\dagger \mathbf{D}^{(\ell)} = \mathbf{E}_\ell \Longleftrightarrow | \left(\mathbf{D}^{(\ell)}\right)^\dagger \mathbf{D}^{(\ell)} = \mathbf{E}_\ell \Longleftrightarrow | ||
| Line 205: | Line 307: | ||
\big(D^{(\ell)}_{mm'}\big)^* D^{(\ell)}_{m m''} = \delta_{m' m''}, | \big(D^{(\ell)}_{mm'}\big)^* D^{(\ell)}_{m m''} = \delta_{m' m''}, | ||
</math> | </math> | ||
where '''E'''<sub>'' | where '''E'''<sub>''ℓ''</sub> is the 2''ℓ''+1 dimensional [[identity matrix]]. | ||
From this unitarity follows the following useful invariance | From this unitarity follows the following useful invariance | ||
:<math> | :<math> | ||
| Line 219: | Line 321: | ||
D^{(\ell)}(\mathbf{R})_{m'm}. | D^{(\ell)}(\mathbf{R})_{m'm}. | ||
</math> | </math> | ||
Let θ and φ be the spherical polar angles of '''r''', then | Let θ and φ be the spherical polar angles of '''r''', then as is shown [[Spherical polar coordinates#Unit vectors|here]], | ||
:<math> | :<math> | ||
\left[ | \left[ | ||
| Line 252: | Line 354: | ||
:<math> | :<math> | ||
C^m_\ell(\hat{\mathbf{r}}) = \sum_{m'=-\ell}^{\ell} \delta_{m',0} | C^m_\ell(\hat{\mathbf{r}}) = \sum_{m'=-\ell}^{\ell} \delta_{m',0} | ||
D^{(\ell)}(\varphi,\theta,0)_{mm'}^* | D^{(\ell)}(\varphi,\theta,0)_{mm'}^*. | ||
</math> | |||
Hence, | |||
:<math> | |||
C^m_\ell(\theta,\varphi)^* = D^{(\ell)}(\varphi,\theta,0)_{m0}. | |||
</math> | </math> | ||
==Completeness of spherical harmonics== | ==Completeness of spherical harmonics== | ||
The spherical harmonics are orthogonal and it can be shown that they are complete in the least squares sense for functions ''f'' of θ and φ. That is, the square of the "distance" between ''f'' and the expansion | The spherical harmonics are orthogonal and it can be shown that they are complete in the least squares sense for functions ''f'' of θ and φ. That is, the square of the "distance" between ''f'' and the expansion | ||
| Line 269: | Line 376: | ||
c_{\ell,m} = \int_0^\pi \int_0^{2\pi} Y_\ell^m(\theta, \varphi)^* f(\theta,\varphi)\; \sin\theta\; d\theta\, d\varphi. | c_{\ell,m} = \int_0^\pi \int_0^{2\pi} Y_\ell^m(\theta, \varphi)^* f(\theta,\varphi)\; \sin\theta\; d\theta\, d\varphi. | ||
</math> | </math> | ||
The proof of the completeness follows from the facts that the exponential functions of φ are complete, as is known from [[Fourier theory]] and that the | The proof of the completeness follows from the facts that the exponential functions of φ are complete, as is known from [[Fourier theory]] and that the associated Legendre differential equation is of the [[Sturm-Liouville]] type. In quantum mechanics one expresses this by stating that the associated Legendre equation is an eigenvalue equation of a Hermitian operator. | ||
Alternatively one can invoke the [[Peter-Weyl theorem]], from which follows that the Wigner ''D''-matrices are complete, as the rotation [[group]] SO(3) is [[compact]]. In general Wigner ''D''-matrices depend on three rotation angles (for instance [[Euler angles]]). Application of the completeness of the ''D''-matrices to functions that do not depend on one of the three angles proves the completeness of spherical harmonics, while noting the relation between the spherical harmonics and the ''D''-matrices pointed out earlier in this article. | Alternatively one can invoke the [[Peter-Weyl theorem]], from which follows that the Wigner ''D''-matrices are complete, as the rotation [[group]] SO(3) is [[compact]]. In general Wigner ''D''-matrices depend on three rotation angles (for instance [[Euler angles]]). Application of the completeness of the ''D''-matrices to functions that do not depend on one of the three angles proves the completeness of spherical harmonics, while noting the relation between the spherical harmonics and the ''D''-matrices pointed out earlier in this article. | ||
| Line 299: | Line 406: | ||
</math> | </math> | ||
which proves the spherical harmonic addition theorem. | which proves the spherical harmonic addition theorem. | ||
As a corollary we find ''Unsöld's theorem''<ref>A. Unsöld, Ann. der Physik, vol. '''82''', p.355 (1927)</ref> | |||
:<math> | |||
1 = \frac{4\pi}{2\ell+1} \sum_{m=-\ell}^\ell Y_\ell^m(\hat{\mathbf{r}})^*\; Y_\ell^m(\hat{\mathbf{r}}), | |||
</math> | |||
by simply taking <math>\scriptstyle \hat{\mathbf{r}}_1 = \hat{\mathbf{r}}_2 = \hat{\mathbf{r}}</math>. | |||
==Gaunt series== | ==Gaunt series== | ||
| Line 337: | Line 450: | ||
\end{pmatrix}, | \end{pmatrix}, | ||
</math> | </math> | ||
where the quantity with three | where the quantity with three zeros in the bottom row is also a 3j-symbol. | ||
==Real form== | ==Real form== | ||
| Line 370: | Line 483: | ||
</math> | </math> | ||
The real functions are sometimes referred to as ''tesseral harmonics'', see Whittaker and Watson<ref name="Whittaker" /> p. 392 for an explanation of this name. | The real functions are sometimes referred to as ''tesseral harmonics'', see Whittaker and Watson<ref name="Whittaker" /> p. 392 for an explanation of this name. | ||
==References== | ==References== | ||
<references /> | <references />[[Category:Suggestion Bot Tag]] | ||
Latest revision as of 06:00, 21 October 2024
- See Spherical harmonics/Catalogs for a table of spherical harmonics through ℓ = 4.
Spherical harmonics are functions arising in physics and mathematics when spherical polar coordinates (coordinates r, θ and φ that locate a point in space) are used in investigating physical problems in three dimensions. The functions appear in physical problems with (near-) spherical symmetry, indeed, in the same kind of physical problems where spherical polar coordinates are preferred over other coordinate systems such as Cartesian or cylinder coordinates.
The name "spherical harmonics" was first used by William Thomson (Lord Kelvin) and Peter Guthrie Tait in their 1867 Treatise on Natural Philosophy.[1] The term harmonic function was coined earlier by William Thomson for solutions of the Laplace equation, ∇²V = 0, and as the spherical harmonic functions appear as the solution of the Laplace equation in spherical polar coordinates, their name followed immediately. In German the functions are called "Kugelfunktionen" (literally sphere functions), and in French they are known as "fonctions harmoniques sphériques", which is equivalent to their English name.
In quantum mechanics spherical harmonics appear as eigenfunctions of (squared) orbital angular momentum. Spherical harmonics are ubiquitous in atomic and molecular physics. Further, they are important in the representation of the gravitational and magnetic fields of planetary bodies, the characterization of the cosmic microwave background radiation, the rotation-invariant description of 3D shapes in computer graphics, the description of electrical potentials due to charge distributions, and in certain types of fluid motion.
It can be shown that the spherical harmonics, almost always written as , form an orthogonal and complete set (a basis of a Hilbert space) of functions of the spherical polar angles, θ and φ, with ℓ and m indicating degree and order of the function. This implies that the harmonics can be used to describe a function of θ and φ in the form of a linear expansion; the expansion coefficients may be used as linear regression parameters, which means that they may be chosen such that the original and expanded function "resemble" each other as closely as possible. The more spherical symmetry the original function possesses, the shorter the expansion and the fewer fit (regression) parameters have to be determined.
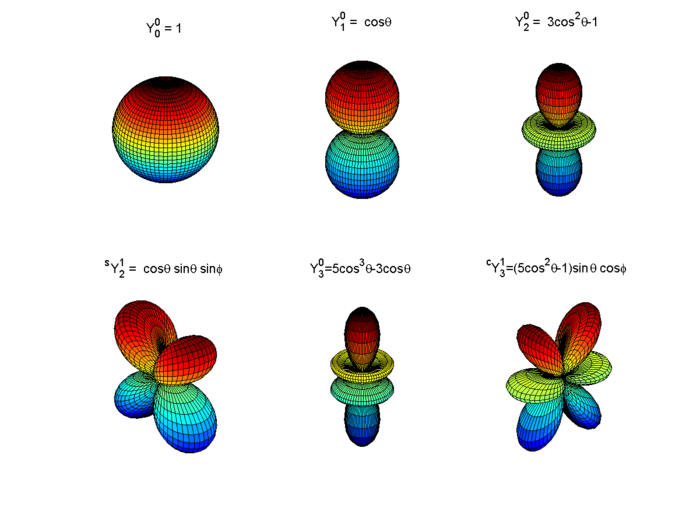
Some illustrative images of real spherical harmonics
Polar plots are shown of a few low-order real spherical harmonics (functions of θ and φ) to be defined in this article. The plots show clearly the nodal planes of the functions. The absolute values are meaningless because the functions are not normalized and accordingly the normalization factors are omitted from their definitions.
Definition of complex spherical harmonics
The notation will be reserved for the complex-valued functions normalized to unity. It is convenient to introduce first non-normalized functions that are proportional to the . Several definitions are possible, we start with one that is common in quantum mechanically oriented texts. The spherical polar angles are the colatitude angle θ and the longitudinal (azimuthal) angle φ. The numbers ℓ and m are integral numbers and ℓ is positive or zero.
where is a (phaseless) associated Legendre function. The m dependent phase is known as the Condon & Shortley phase:
An alternative definition uses the fact that the associated Legendre functions can be defined (via the Rodrigues formula) for negative m,
The two definitions obviously agree for positive and zero m, but for negative m this is less apparent. It is also not immediately clear that the choices of phases yield the same function. However, below we will see that the definitions agree for negative m as well. Hence, for all ℓ ≥ 0,
Complex conjugation
Noting that the associated Legendre function is real and that
we find for the complex conjugate of the spherical harmonic in the first definition
Complex conjugation gives for the functions of positive m in the second definition
Use of the following non-trivial relation (that does not depend on any choice of phase):
gives
Since the two definitions of spherical harmonics coincide for positive m and complex conjugation gives in both definitions the same relation to functions of negative m, it follows that the two definitions agree. From here on we drop the tilde and assume both definitions to be simultaneously valid.
Note
If the m-dependent phase would be dropped in both definitions, the functions would still agree for non-negative m. However, the first definition would satisfy
whereas the second would still satisfy
from which follows that the functions would differ in phase for negative m.
Normalization
It can be shown that
The integral over φ gives 2π and a Kronecker delta on m and m′. Thus, for the integral over θ it suffices to consider the case m = m'. The necessary integral is given here. The (non-unit) normalization of is known as Racah's normalization or Schmidt's semi-normalization. It is often more convenient than unit normalization. Unit normalized functions are defined as follows
Condon-Shortley phase
One source of confusion with the definition of the spherical harmonic functions concerns the phase factor. In quantum mechanics the phase, introduced above, is commonly used. It was introduced by Condon and Shortley.[2] In the quantum mechanics community, it is common practice to either include this phase factor in the definition of the associated Legendre functions, or to prefix it to the definition of the spherical harmonic functions, as done above. There is no requirement to use the Condon-Shortley phase in the definition of the spherical harmonic functions, but including it can simplify some quantum mechanical operations, especially the application of raising and lowering operators. The geodesy and magnetics communities never include the Condon-Shortley phase factor in their definitions of the spherical harmonic functions.
Properties
- For m ≠ 0 the associated Legendre function contains the factor (1−x2) and the ordinary Legendre polynomial Pn(1) = 1. So,
- The regular solid harmonics rℓYℓm are homogeneous of degree ℓ in the components x, y, and z of r, so that inversion r → −r gives the factor (−1)ℓ for the regular solid harmonics. Inversion of spherical polar coordinates: r → r, θ → π−θ, and φ → π+φ. So,
- Reflection in the x-y plane:
Eigenfunctions of orbital angular momentum
In quantum mechanics the following operator, the orbital angular momentum operator, appears frequently
where the cross stands for the cross product of the position vector r and the gradient ∇; ℏ is Planck's constant divided by 2π. The components of L satisfy the angular momentum commutation relations:
where εijk is the Levi-Civita symbol. These relations define a Lie algebra, as discussed later in this article. In angular momentum theory it is shown that these commutation relations are sufficient to prove that L² has eigenvalues ℓ(ℓ+1),
where ℓ is a natural number. From here on we take ℏ equal to unity (this is part of the system of atomic units). The operator L² expressed in spherical polar coordinates is,
The eigenvalue equation can be simplified by separation of variables. We substitute
into the eigenvalue equation. After dividing out Ψ and multiplying with sin²θ we get
In the spirit of the method of separation of variables, we put the terms in square brackets equal to plus and minus the same constant, respectively. Without loss of generality we take m² as this constant (m can be complex) and consider
This has the solutions
The requirement that exp[i m (φ + 2π)] = exp[i m φ] gives that m is integral. Substitution of this result into the eigenvalue equation gives
Upon writing x = cos θ the equation becomes the associated Legendre equation
This equation has two classes of solutions: the associated Legendre functions of the first and second kind. The functions of the second kind are non-regular for x = ±1 and do not concern us further. The functions of the first kind are the associated Legendre functions:
It follows that
The eigenvalue equation does not establish phase and normalization, so that these must be imposed separately. This was done earlier in this article.
Finally, noting that
we summarize the action of the components of orbital angular momentum on spherical harmonics:
Laplace equation
The Laplace equation ∇² Ψ = 0 reads in spherical polar coordinates
Clearly, this can be rewritten as
Making the Ansatz Ψ = R(r) Ymℓ the equation becomes
where we divided out . Inserting the following functions
shows that these functions are solutions. They give rise to functions known as regular and irregular solid harmonics. See solid harmonics for more details.
Finally, it is evident that
because .
Lie algebra
Consider the infinitesimal rotation from which finite rotations can be generated.[3] For example, a rotation by angle α about the z-axis is described by the matrix:
where the form following the arrow applies for very small angles α. The matrix Rz is called the generator of the z-rotation. The factor i is introduced so the finite rotation can be expressed in terms of this generator as a simple exponential:
as can be verified using the Taylor series:
If the three coordinate axes are labeled {i, j, k } and the infinitesimal rotations about each of these axes are labeled {Ri, Rj, Rk}, then these generators of infinitesimal rotations obey the commutation relations:[4]
for any choices of subscripts. Here εijk is the Levi-Civita symbol mentioned above. The commutation relations express the fact that the order (sequence) of rotations matters.
Suppose these commutation relations now are viewed as applying in general, and while still considered as connected to rotations in three dimensional space, the question is opened as to what general mathematical objects might satisfy these rules. A set of symbols with a defined sum and a product taken as a commutator of the symbols is called a Lie algebra. [5] In particular, one can construct sets of square matrices of various dimensions that satisfy these commutation rules; each set is a so-called representation of the rules. One finds that there are many such sets, but they can be sorted into two kinds: irreducible and reducible. The reducible sets of matrices can be shown to be equivalent to matrices with smaller irreducible matrices down the diagonal, so that the rules are satisfied within these smaller constituent matrices, and the entire matrix is not essential. The irreducible sets cannot be arranged this way.[6]
The matrices can be viewed as acting upon vectors in an abstract space. For example, a space with an odd number of dimensions (2ℓ+1) can be constructed from the spherical harmonics Yℓm, and their transformations under infinitesimal rotations. The Yℓm depend upon the angles θ,φ describing orientation in ordinary three-dimensional space, but infinitesimal rotations of these arguments mix up the Yℓm in a fashion described by irreducible matrices of dimension (2ℓ+1) that satisfy the commutation relations.[7]
The construction of irreducible matrices of any dimension at all is done as follows. If the generator of an infinitesimal rotation is labeled J, then the basis vectors in this space can be labeled by the integers j and m where m is restricted to the values { −j, −j+1, ... , j−1, j }. Denoting a basis vector by |j, m⟩, one finds:
Here Jz generates an infinitesimal rotation about a direction chosen as the z-axis, and J2 = Jx2 + Jy2 + Jz2 is the so-called Casimir operator[8]. In particular, these equations recover the Pauli spin matrices in two dimensions and the infinitesimal transformations of the Yℓm in (2ℓ+1) dimensions.[9] See the development in Eigenfunctions of orbital angular momentum earlier in this article.
Connection with 3D full rotation group
The group of proper (no reflections) rotations in three dimensions is SO(3). It consists of all 3 x 3 orthogonal matrices with unit determinant. A unit vector is uniquely determined by two spherical polar angles and conversely. Hence we write
Let R be a unimodular (unit determinant) orthogonal matrix, then we define a rotation operator by
The inverse matrix appears here (acting on a column vector) in order to assure that this map of rotation matrices to rotation operators is a group homomorphism. Since this point was discussed at some length in Wigner's famous book on group theory,[10] it is known as Wigner's convention. Some authors omit the inverse on the rotation and find accordingly that the map from matrices to operators is antihomomorphic (i.e., multiplication of operators and matrices is in mutually reversed order).
It can be shown that the rotation operator is an exponential operator in the components of the orbital angular momentum operator L. It can also be shown that the action of these operators on the spherical harmonics do no change ℓ. That is, the linear space spanned by 2ℓ+1 spherical harmonics of same ℓ and different m is invariant under L, and therefore also under rotations,
The square 2ℓ+1 dimensional matrix that appears here is known as Wigner's D-matrix. Obviously, the set of matrices of fixed ℓ form a representation of the group SO(3). It can be shown that they form an irreducible representation of this group. The rotation operator is unitary and the spherical harmonics are orthonormal, hence the Wigner rotation matrix is a unitary matrix:
where Eℓ is the 2ℓ+1 dimensional identity matrix. From this unitarity follows the following useful invariance
Connection with Wigner D-matrices
The rotation of spherical harmonics may be rewritten as follows (where we introduce the Racah normalized functions):
Let θ and φ be the spherical polar angles of r, then as is shown here,
Substitution of this rotation, use of group homomorphism and unitarity of D-matrices,
and the fact that spherical harmonics of zero θ give a Kronecker delta on m, we get a relation between spherical harmonics and Wigner D-matrices,
Hence,
Completeness of spherical harmonics
The spherical harmonics are orthogonal and it can be shown that they are complete in the least squares sense for functions f of θ and φ. That is, the square of the "distance" between f and the expansion
can be made arbitrarily small for sufficiently large N. It is common to write somewhat loosely
It is known from Hilbert space theory that the expansion (Fourier) coefficients are given by
The proof of the completeness follows from the facts that the exponential functions of φ are complete, as is known from Fourier theory and that the associated Legendre differential equation is of the Sturm-Liouville type. In quantum mechanics one expresses this by stating that the associated Legendre equation is an eigenvalue equation of a Hermitian operator.
Alternatively one can invoke the Peter-Weyl theorem, from which follows that the Wigner D-matrices are complete, as the rotation group SO(3) is compact. In general Wigner D-matrices depend on three rotation angles (for instance Euler angles). Application of the completeness of the D-matrices to functions that do not depend on one of the three angles proves the completeness of spherical harmonics, while noting the relation between the spherical harmonics and the D-matrices pointed out earlier in this article.
Spherical harmonic addition theorem
The spherical harmonic addition theorem reads
There are two proofs: a short one, referred to by Whittaker and Watson[11] (p. 395) as a "physical proof", and a long analytic proof.[12]
We skip the analytic proof and outline the physical proof. Under a simultaneous rotation R of two vectors the angle between them is not changed,
because RTR is equal to the 3 × 3 identity matrix. Choose the rotation R such that the rotated unit vector coincides with the z-axis, and use that the sum over m in the following is a rotation invariant (see earlier in this article)
The angle θ1 is the colatitude (polar) angle of the rotated vector r1 and hence is the angle with the rotated vector r2, which lies along the z-axis. Since the angle between the two vectors is invariant under rotation we have
which proves the spherical harmonic addition theorem.
As a corollary we find Unsöld's theorem[13]
by simply taking .
Gaunt series
Since the spherical harmonics are complete and orthonormal, we can expand a binary product of spherical harmonics again in spherical harmonics. This gives the Gaunt series,
with
This double integral is called a Gaunt[14] coefficient. By the Wigner-Eckart theorem it is proportional to the 3j-symbol
The 3j-symbol is zero unless
These conditions constrain the sum over L in the Gaunt series and remove the sum over M. In total the Gaunt coefficient is
where the quantity with three zeros in the bottom row is also a 3j-symbol.
Real form
The following expression defines real spherical harmonics of cosine and sine type respectively:
and for m = 0:
Since the transformation is by a unitary matrix the normalization of the real and the complex spherical harmonics is the same. By definition, for m > 0 we have the phaseless expressions
The real functions are sometimes referred to as tesseral harmonics, see Whittaker and Watson[11] p. 392 for an explanation of this name.
References
- ↑ See N. M. Ferrers, An Elementary Treatise on Spherical Harmonics, MacMillan, 1877 (London) p. 3 Online.
- ↑ E. U. Condon and G. H. Shortley,The Theory of Atomic Spectra, Cambridge University Press, Cambridge UK (1935).
- ↑ This development is close to that found in David McMahon (2008). “The special orthogonal group SO(N)”, Quantum field theory demystified. McGraw-Hill Professional, pp. 58 ff. ISBN 0071543821.
- ↑ Kurt Gottfried, Tung-mow Yan (2003). Quantum mechanics: fundamentals, 2nd ed. Springer, p. 77. ISBN 0387955763.
- ↑ For a mathematical discussion see R. Mirman (1997). “§X.7 Angular momentum operators and their algebra”, Group Theory: An Intuitive Approach. World Scientific Publishing Company, pp. 292 ff. ISBN 9810233655. Matrices satisfying the commutation rules are called a matrix representation of the Lie algebra. See BG Adams, J Cizek, J Paldus (1987). “§2.2 Matrix representation of a Lie algebra”, Arno Böhm et al.: Dynamical groups and spectrum generating algebras, vol. 1, Reprint of article in Advances in Quantum Chemistry, vol. 19, Academic Press, 1987. World Scientific, pp. 114 ff. ISBN 9971501473.
- ↑ For a discussion see Hermann Weyl (1950). “Chapter IV A §1 The representation induced in system space by the rotation group”, The theory of groups and quantum mechanics, Reprint of 1932 ed. Courier Dover Publications, pp. 185 ff. ISBN 0486602699. , or John M. Brown, Alan Carrington (2003). “§5.2.4 Representations of the rotation group”, Rotational spectroscopy of diatomic molecules. Cambridge University Press, pp. 143 ff. ISBN 0521530784.
- ↑ Jean Hladik (1999). “§3.3.2 Spherical harmonics”, Spinors in physics. Springer, pp. 83ff. ISBN 0387986472.
- ↑ Yvette Kosmann-Schwarzbach (2009). “§3.2: The Casimir operator”, Groups and Symmetries: From Finite Groups to Lie Groups, Stephanie Frank Singer translation. Springer, pp. 99 ff. ISBN 0387788654.
- ↑ For example, see John M. Blatt, Victor F. Weisskopf (1991). Theoretical nuclear physics, Reprint of 1979 Springer-Verlag ed. Courier Dover Publications, p. 782. ISBN 0486668274.
- ↑ E. P. Wigner, Gruppentheorie und ihre Anwendungen auf die Quantenmechanik der Atomspektren, Vieweg Verlag, Braunschweig (1931). Translated into English: J. J. Griffin, Group Theory and its Application to the Quantum Mechanics of Atomic Spectra, Academic Press, New York (1959).
- ↑ 11.0 11.1 E. T. Whittaker and G. N. Watson, A Course of Modern Analysis, Cambridge UP, Cambridge UK, 4th edition (1927)
- ↑ H. Margenau and G. M. Murphy, The Mathematics of Physics and Chemistry, 2nd edition, Van Nostrand, New York (1956), pp. 109-113. This proof involves a contour integral and several ordinary integrals
- ↑ A. Unsöld, Ann. der Physik, vol. 82, p.355 (1927)
- ↑ J. A. Gaunt, Phil. Trans. Roy. Soc. (London) vol A228, p. 151 (1929)


![{\displaystyle C_{\ell }^{m}(\theta ,\varphi )\equiv i^{m+|m|}\;\left[{\frac {(\ell -|m|)!}{(\ell +|m|)!}}\right]^{1/2}P_{\ell }^{(|m|)}(\cos \theta )e^{im\varphi },\qquad -\ell \leq m\leq \ell ,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a82104a043e1c9c5988a0fc93f78f5f1eecd4d74)


![{\displaystyle {\tilde {C}}_{\ell }^{m}(\theta ,\varphi )\equiv (-1)^{m}\left[{\frac {(\ell -m)!}{(\ell +m)!}}\right]^{1/2}P_{\ell }^{(m)}(\cos \theta )e^{im\varphi },\qquad -\ell \leq m\leq \ell ,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5803fa5ca760d948bbe8592c48a96e16b087ef07)


![{\displaystyle C_{\ell }^{m}(\theta ,\varphi )^{*}=(-1)^{m}\,i^{-m+|m|}\;\left[{\frac {(\ell -|m|)!}{(\ell +|m|)!}}\right]^{1/2}P_{\ell }^{(|m|)}(\cos \theta )e^{-im\varphi }=(-1)^{m}C_{\ell }^{-m}(\theta ,\varphi ).}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4bbf6b02a48607296d6be9aa6ae4993b6f5c0505)
![{\displaystyle {\tilde {C}}_{\ell }^{|m|}(\theta ,\varphi )^{*}\equiv (-1)^{m}\left[{\frac {(\ell -|m|)!}{(\ell +|m|)!}}\right]^{1/2}P_{\ell }^{(|m|)}(\cos \theta )e^{-i|m|\varphi }.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/65e82fcce244e2a1878990c4383c9cc00c4a407e)

![{\displaystyle {\tilde {C}}_{\ell }^{|m|}(\theta ,\varphi )^{*}=\left[{\frac {(\ell +|m|)!}{(\ell -|m|)!}}\right]^{1/2}P_{\ell }^{(-|m|)}(\cos \theta )e^{-i|m|\varphi }=(-1)^{m}{\tilde {C}}_{\ell }^{-|m|}(\theta ,\varphi ).}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8350e6a017e2c920f8f8e866b2f5602f293da23e)









![{\displaystyle [L_{i},L_{j}]=i\hbar \sum _{j=1}^{3}\epsilon _{ijk}L_{k},\qquad i,j,k=x,y,z,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1800a82ad7faeeeea7435d1b98d4e8dc89e02ae7)

![{\displaystyle L^{2}=-\left[{\frac {1}{\sin \theta }}{\frac {\partial }{\partial \theta }}\sin \theta {\frac {\partial }{\partial \theta }}+{\frac {1}{\sin ^{2}\theta }}{\frac {\partial ^{2}}{\partial \varphi ^{2}}}\right].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6a9b2dfc458ade3279c0d296f8f4f2dab3cfceeb)

![{\displaystyle \left[{\frac {1}{\Theta (\theta )}}\sin \theta {\frac {\partial }{\partial \theta }}\sin \theta {\frac {\partial \Theta (\theta )}{\partial \theta }}+\ell (\ell +1)\sin ^{2}\theta \right]+\left[{\frac {1}{\Phi (\varphi )}}{\frac {\partial ^{2}\Phi (\varphi )}{\partial \varphi ^{2}}}\right]=0.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/34efb19553b65cbe0a6bf8f964dd105337175795)


![{\displaystyle \left[{\frac {1}{\sin \theta }}{\frac {\partial }{\partial \theta }}\sin \theta {\frac {\partial \Theta (\theta )}{\partial \theta }}+\ell (\ell +1)-{\frac {m^{2}}{\sin ^{2}\theta }}\right]\Theta (\theta )=0.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/95bbdcd3ba96f9d538a692b374e14e79b1e561f6)
![{\displaystyle (1-x^{2}){\frac {d^{2}\Theta }{dx^{2}}}-2x{\frac {d\Theta }{dx}}+\left[\ell (\ell +1)-{\frac {m^{2}}{1-x^{2}}}\right]\Theta =0.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/87e659638ef64b417c75c67fecf8ff557c964d44)























![{\displaystyle \left[{\begin{pmatrix}\cos \varphi &-\sin \varphi &0\\\sin \varphi &\cos \varphi &0\\0&0&1\\\end{pmatrix}}{\begin{pmatrix}\cos \theta &0&\sin \theta \\0&1&0\\-\sin \theta &0&\cos \theta \\\end{pmatrix}}\right]^{-1}{\begin{pmatrix}\cos \varphi \sin \theta \\\sin \varphi \sin \theta \\\cos \theta \end{pmatrix}}={\begin{pmatrix}0\\0\\1\end{pmatrix}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e43f5530fe01ce249e8d5f1c5b5671c5e597f0ce)




















![{\displaystyle {\begin{aligned}^{c}Y_{\ell }^{m}=&{\sqrt {\frac {2\ell +1}{2\pi }}}\left[{\frac {(\ell -m)!}{(\ell +m)!}}\right]^{1/2}P_{\ell }^{(m)}(\cos \theta )\cos m\varphi ,\\^{s}Y_{\ell }^{m}=&{\sqrt {\frac {2\ell +1}{2\pi }}}\left[{\frac {(\ell -m)!}{(\ell +m)!}}\right]^{1/2}P_{\ell }^{(m)}(\cos \theta )\sin m\varphi .\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e379ea6278aad0a0e30a9ce5fabf2b76bd174f25)