Pressure: Difference between revisions
imported>Milton Beychok m (→Absolute pressure versus gauge pressure: Expanded discusion of pressure modifiers.) |
mNo edit summary |
||
| (5 intermediate revisions by 2 users not shown) | |||
| Line 23: | Line 23: | ||
{{pressure}}<br/> | {{pressure}}<br/> | ||
'''About the torr:''' There is no consensus in the technical literature about whether the name of the torr should be "Torr" or "torr". Nor is there any consensus about whether the symbol for that unit of pressure should be "Torr" or "torr". Both the [[United Kingdom]]'s [[National Physical Laboratory]] (see [http://www.npl.co.uk/reference/faqs/pressure-units Pressure Units]) and [[New Zealand]]'s [[Measurement Standards Laboratory]] (see [http://msl.irl.cri.nz/sites/all/files/training-manuals/TG19-July-2009.pdf Barometric Pressure Units]) use "torr" as the name and as the symbol. An extensive search of the website of the [[U.S.]] [[National Institute of Standards and Technology]] found no such clear-cut definitions. Therefore, this table uses "torr" as both the name and the symbol. | '''About the torr:''' There is no consensus in the technical literature about whether the name of the torr should be "Torr" or "torr". Nor is there any consensus about whether the symbol for that unit of pressure should be "Torr" or "torr". Both the [[United Kingdom]]'s [[National Physical Laboratory]] (see [http://www.npl.co.uk/reference/faqs/pressure-units Pressure Units]) and [[New Zealand]]'s [[Measurement Standards Laboratory]] (see [http://msl.irl.cri.nz/sites/all/files/training-manuals/TG19-July-2009.pdf Barometric Pressure Units]) use "torr" as the name and as the symbol. An extensive search of the website of the [[United States of America|U.S.]] [[National Institute of Standards and Technology]] found no such clear-cut definitions. Therefore, this table uses "torr" as both the name and the symbol. | ||
== Absolute pressure versus gauge pressure == | == Absolute pressure versus gauge pressure == | ||
| Line 33: | Line 33: | ||
An example of the difference is between gauge and absolute pressure is the air pressure in a vehicle tire. A tire [[pressure gauge]] might read 220 kPa as the gauge pressure, but that means the pressure is 220 kPa above atmospheric pressure. Since atmospheric pressure at sea level is about 101 kPa, the absolute pressure in the tire is therefore about 321 kPa. | An example of the difference is between gauge and absolute pressure is the air pressure in a vehicle tire. A tire [[pressure gauge]] might read 220 kPa as the gauge pressure, but that means the pressure is 220 kPa above atmospheric pressure. Since atmospheric pressure at sea level is about 101 kPa, the absolute pressure in the tire is therefore about 321 kPa. | ||
In technical writing, this would be written as ''a gauge pressure of 220 kPa'' or as ''an absolute pressure of 321 kPa''. Where space is limited, such as on pressure gauge dials, table headings or graph labels, the use of a modifier in parentheses, such as ''kPa (gauge)'' and ''kPa (absolute)'' or ''kPa-gauge'' and ''kPa-absolute'', is strongly encouraged.<ref name=NPL/><ref name=Jones/> Gauge pressure is | In technical writing, this would be written as ''a gauge pressure of 220 kPa'' or as ''an absolute pressure of 321 kPa''. Where space is limited, such as on pressure gauge dials, table headings or graph labels, the use of a modifier in parentheses, such as ''kPa (gauge)'' and ''kPa (absolute)'' or ''kPa-gauge'' and ''kPa-absolute'', is strongly encouraged.<ref name=NPL/><ref name=Jones/> Gauge pressure is also sometimes spelled ''gage pressure''. This discussion of modifiers also applies to other pressure units such as bar, torr, atmosphere, etc. | ||
==Negative or vacuum pressures== | ==Negative or vacuum pressures== | ||
While pressures are generally positive, when describing a system operating at a pressure below | While pressures are generally positive, when describing a system operating at a pressure below atmospheric pressure (a [[Vacuum (partial)|partial vacuum]]), the pressure (as shown in Figure 1) may be expressed in terms of how much it is below atmospheric pressure or in terms of how much it is above a complete vacuum. For example, if the atmospheric pressure is 101 kPa, point B in Figure 1 might be expressed as being 45 kPa of vacuum, meaning it is 45 kPa below atmospheric pressure. It might also be expressed as 56 kPa (absolute) meaning that it is 56 kPa above zero pressure. In technical writing, it is very important to make clear how any pressures below atmospheric pressure are expressed. | ||
A statement such as ''the system operates at a vacuum of 100 torr'' will lead to confusion because it might mean an absolute pressure of 100 torr or a pressure of −100 torr (i.e., 100 torr below an atmospheric pressure of 760 torr). | A statement such as ''the system operates at a vacuum of 100 torr'' will lead to confusion because it might mean an absolute pressure of 100 torr or a pressure of −100 torr (i.e., 100 torr below an atmospheric pressure of 760 torr). | ||
| Line 60: | Line 58: | ||
<ref name=Jones>{{cite book|author=Arnold Ivan Jones and Cornelius Wandmacher|title=Metric Units in Engineering:Going SI|edition=Revised Edition|publisher=American Society of Civil Engineers|year=2007|pages=page 147|id=ISBN 0-7844-0070-9}}</ref> | <ref name=Jones>{{cite book|author=Arnold Ivan Jones and Cornelius Wandmacher|title=Metric Units in Engineering:Going SI|edition=Revised Edition|publisher=American Society of Civil Engineers|year=2007|pages=page 147|id=ISBN 0-7844-0070-9}}</ref> | ||
}} | }}[[Category:Suggestion Bot Tag]] | ||
Latest revision as of 16:01, 6 October 2024
Pressure (symbol: p) is the force applied over an area in a direction perpendicular to the surface of the area.
Pressure is a natural physical phenomenon. It is a scalar quantity and a fundamental parameter in thermodynamics.
Mathematical definition
Mathematically, pressure may be expressed as:[1]
where:
- is the pressure
- is the perpendicular force
- is the area.
The SI unit for pressure is the pascal (Pa), equal to one newton per square metre (N·m-2 or kg·m-1·s-2). It was given that SI name in 1971. Before that, pressure in SI was expressed simply as N/m2.
Various units of pressure
| pascal (Pa) |
bar (bar) |
atmosphere (atm) |
torr (torr) |
pound-force per square inch (psi) |
kilogram-force per square centimeter (kgf/cm2) | |
|---|---|---|---|---|---|---|
| 1 Pa | ≡ 1 N/m2 | 10−5 | 9.8692×10−6 | 7.5006×10−3 | 145.04×10−6 | 1.01972×10−5 |
| 1 bar | 100,000 | ≡ 106 dyn/cm2 | 0.98692 | 750.06 | 14.504 | 1.01972 |
| 1 atm | 101,325 | 1.01325 | ≡ 1 atm | 760 | 14.696 | 1.03323 |
| 1 torr | 133.322 | 1.3332×10−3 | 1.3158×10−3 | ≡ 1 torr ≈ 1 mmHg |
19.337×10−3 | 1.35951×10−3 |
| 1 psi | 6,894.76 | 68.948×10−3 | 68.046×10−3 | 51.715 | ≡ 1 lbf/in2 | 7.03059×10−2 |
| 1 kgf/cm2 | 98,066.5 | 0.980665 | 0.967838 | 735.5576 | 14.22357 | ≡ 1 kgf/cm2 |
Example reading: 1 Pa = 1 N/m2 = 10−5 bar = 9.8692×10−6 atm = 7.5006×10−3 torr, etc.
Note: mmHg is an abbreviation for millimetre of mercury
About the torr: There is no consensus in the technical literature about whether the name of the torr should be "Torr" or "torr". Nor is there any consensus about whether the symbol for that unit of pressure should be "Torr" or "torr". Both the United Kingdom's National Physical Laboratory (see Pressure Units) and New Zealand's Measurement Standards Laboratory (see Barometric Pressure Units) use "torr" as the name and as the symbol. An extensive search of the website of the U.S. National Institute of Standards and Technology found no such clear-cut definitions. Therefore, this table uses "torr" as both the name and the symbol.
Absolute pressure versus gauge pressure
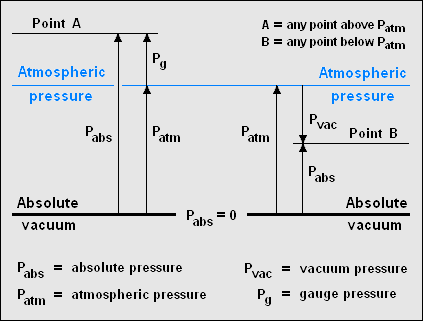
Bourdon tube pressure gauges, vehicle tire gauges and many other types of pressure gauges are zero referenced to atmospheric pressure, which means that they measure the pressure above atmospheric pressure. However, absolute pressures are zero referenced to a complete vacuum. Thus, the absolute pressure of any system is the gauge pressure of the system plus the local atmospheric or ambient pressure. Figure 1 illustrates the relationship between gauge and absolute pressure for any pressures above atmospheric pressure.
An example of the difference is between gauge and absolute pressure is the air pressure in a vehicle tire. A tire pressure gauge might read 220 kPa as the gauge pressure, but that means the pressure is 220 kPa above atmospheric pressure. Since atmospheric pressure at sea level is about 101 kPa, the absolute pressure in the tire is therefore about 321 kPa.
In technical writing, this would be written as a gauge pressure of 220 kPa or as an absolute pressure of 321 kPa. Where space is limited, such as on pressure gauge dials, table headings or graph labels, the use of a modifier in parentheses, such as kPa (gauge) and kPa (absolute) or kPa-gauge and kPa-absolute, is strongly encouraged.[2][3] Gauge pressure is also sometimes spelled gage pressure. This discussion of modifiers also applies to other pressure units such as bar, torr, atmosphere, etc.
Negative or vacuum pressures
While pressures are generally positive, when describing a system operating at a pressure below atmospheric pressure (a partial vacuum), the pressure (as shown in Figure 1) may be expressed in terms of how much it is below atmospheric pressure or in terms of how much it is above a complete vacuum. For example, if the atmospheric pressure is 101 kPa, point B in Figure 1 might be expressed as being 45 kPa of vacuum, meaning it is 45 kPa below atmospheric pressure. It might also be expressed as 56 kPa (absolute) meaning that it is 56 kPa above zero pressure. In technical writing, it is very important to make clear how any pressures below atmospheric pressure are expressed.
A statement such as the system operates at a vacuum of 100 torr will lead to confusion because it might mean an absolute pressure of 100 torr or a pressure of −100 torr (i.e., 100 torr below an atmospheric pressure of 760 torr).
Example effects of pressure
A finger can be pressed against a wall without making a lasting impression; however, the same finger pushing a thumbtack can easily make a small hole in the wall. Although the force applied to the surface is the same, the thumbtack applies more pressure because the point concentrates that force into a smaller area.
Another example is that of a common knife. The flat side of the knife won't cut an apple. But if we use the thin side, it will easily cut the apple. When we use the thin side, more pressure is applied because the surface area is reduced and so it readily cuts the apple.
If one is at the bottom of a deep pool of water, the water pressure will cause pain in one's ears. That pain cannot be relieved by turning one's head. The water's force on your eardrum is always the same, and is always perpendicular to the surface where the eardrum contacts the water.
References
- ↑ IUPAC definition
- ↑ Search Results 1 and 2 (from the website of the National Physics Laboratory, United Kingdom)
- ↑ Arnold Ivan Jones and Cornelius Wandmacher (2007). Metric Units in Engineering:Going SI, Revised Edition. American Society of Civil Engineers, page 147. ISBN 0-7844-0070-9.