Harmonic oscillator (classical)
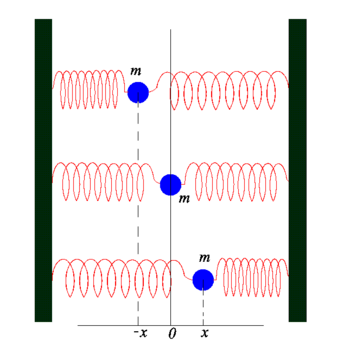
In classical mechanics, a harmonic oscillator appears frequently as a simple model; it describes approximately many different physical phenomena. In its simplest form a harmonic oscillator consists of a mass m on which a force acts that is linear in a displacement from equilibrium. By Hooke's law a spring gives to very good approximation a force that is linear for small displacements and so figure 1 shows a very simple example of a harmonic oscillator. The uppermost mass m feels a force acting to the right equal to f x, where f is Hooke's constant. The mass in the middle is in equilibrium, and the mass at the bottom feels a force to the left equal to −f x. The spring force always acts so as to restore mass back toward its equilibrium position. If the mass is pulled out of equilibrium and then let go, the mass will (in an ideal setup) oscillate for ever. In practice, energy will be lost due to friction of the atoms in the spring, so that the oscillation will die out after some time.
Mathematical description
The motion of the mass as a function of time t may be obtained from Newton's second law (briefly: F = ma). Bringing the force F and the acceleration a (times mass m) to the same side of the equation, the harmonic oscillator equation becomes
We see that the second derivative of x is proportional to −x. There are several functions known to have this property (sine, cosine, exponent with imaginary argument), we choose fairly arbitrarily the cosine as a trial function. The equation is a second order ordinary differential equation and its general solution contains two integration constants. Further the unit of time is undetermined and hence we scale time by a real number ω. In total the trial function is
where the integration constants are to be determined from the initial conditions. Substitution into the Newton equation gives
which fixes . The quantity ω has dimension of frequency (1/time) and is often written as
where ν is the frequency and ω is called angular frequency. As stated, the amplitude and the phase angle φ are integration constants. Often it is possible to choose the zero of time such that ωt + φ = 0, implying that φ is zero:
The amplitude A is determined by how far we move initially (at t = 0) the mass (in figure 1) away from equilibrium
- .
From the form of the cosine function we know that x goes through zero (mass in equilibrium x = 0 in figure 1) at the following points in time:
The displacement is minimum (mass in point x = −A in figure 1, i.e., furthest to the left) for the times
The value of x is maximum (mass in point x = A in figure 1) for
The mass oscillates from x = A to x = −A and back again. The speed of the mass is
- ,
which for the specific values of time is
Physically, after the mass is displaced from equilibrium a distance A to the right, the restoring force fx pushes the mass back toward its equilibrium position, causing it to accelerate to the left. When it reaches equilibrium (x = 0), there is no force acting on it at that instant, but it is moving at speed Aω to the left, and by Newton's first law it persists in its motion moving beyond equilibrium position. Before it is stopped it reaches position −A, and by this time there is a force acting on it again, pulling it back toward equilibrium (to the right in figure 1).
The whole process, known as simple harmonic motion, repeats itself endlessly with an angular frequency given by ω = √f/m. This relation means that the stiffer the springs (i.e., the larger f), the higher the frequency (the faster the oscillations). Making the mass m greater has exactly the opposite effect, slowing the motion down.
One of the most important features of harmonic motion is the fact that the frequency of the motion (ω or ν) depends only on the mass and the stiffness of the spring. It does not depend on the amplitude A of the motion. If the amplitude is increased, the mass moves faster, but the time required for a complete round trip (the argument of the cosine) remains the same. This fact is used in accurate timekeeping.
Harmonic potential
From classical mechanics it is known that the potential energy is minus the force F = −fx integrated over distance:
where the zero of the potential is at the arbitrary point x0 (the lower limit of the integral). When we choose x0 = 0, we find V(x0) = 0, so that V(x) = (f/2)x2.
Making a minor generalization we shift the origin on the x-axis from 0 to the arbitrary point x0, i.e. we make the substitution x → x−x0. Then the harmonic potential becomes the following quadratic function in the displacement x − x0,
It is of interest to show the connection with the Taylor expansion of an arbitrary potential around x0
We truncate the expansion after these three terms. We take V(x0) as zero of potential and assume that x0 is a stationary point of the potential, i.e., that the potential at x0 has a minimum, a maximum, or a point of inflection (saddle point). This means that we assume that
Then the truncated Taylor series shrinks to
If we identify Hooke's (spring) constant thus:
we have found the harmonic potential from the truncated Taylor series. Note that if f > 0, the potential has a minimum at x0 (the stationary point is a minimum).
We went from the force to the potential by integrating over distance. Conversely, given a potential V, it is known from classical mechanics that the corresponding force F is minus the derivative V',
So, one could say that we proved Hooke's law (force linear in displacement) by making (and truncating) a Taylor expansion.
(To be continued)














![{\displaystyle V(x-x_{0})=V(x_{0})\;+\;(x-x_{0})\;\left[{\frac {dV(x)}{dx}}\right]_{x_{0}}\;+\;{\frac {1}{2}}\;(x-x_{0})^{2}\;\left[{\frac {d^{2}V(x)}{dx^{2}}}\right]_{x_{0}}\;+\cdots }](https://wikimedia.org/api/rest_v1/media/math/render/svg/900856fec63c441c2de6b00bc6aad5a8565006e7)
![{\displaystyle \left[{\frac {dV(x)}{dx}}\right]_{x_{0}}=0.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/fc8c1708d52e5198accd21762bcf2d7c38a2f952)
![{\displaystyle V(x-x_{0})={\frac {1}{2}}\;(x-x_{0})^{2}\;\left[{\frac {d^{2}V(x)}{dx^{2}}}\right]_{x_{0}}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/010fb4283193f95163e412103d877a004dee77b2)
![{\displaystyle f=\left[{\frac {d^{2}V(x)}{dx^{2}}}\right]_{x_{0}},}](https://wikimedia.org/api/rest_v1/media/math/render/svg/df26df1571b5e44a3dcf24218a0a8f5b7755841c)
![{\displaystyle F=-V'=-(x-x_{0})\;\left[{\frac {dV(x)}{dx}}\right]_{x_{0}}=-(x-x_{0})\;f.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/848633fec18f75d685e170e49e56c89752c46151)